TUTORIAL · Data Structure
이진 검색 트리 Inorder Succesor
이 튜토리얼에서는 BST에서 노드의 후속 작업을 찾는 방법을 소개합니다.
이 페이지의 내용
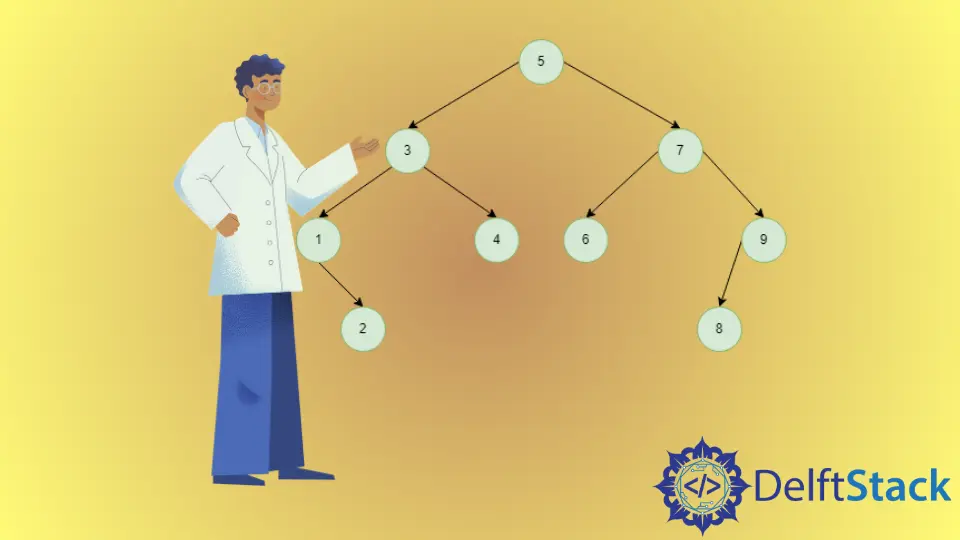
이진 트리의 inorder 후계자는 이진 트리의 inorder traversal에서 다음으로 오는 노드입니다. 따라서 트리 내부의 마지막 노드는NULL입니다. 이진 검색 트리의 순회 순회는 정렬 된 배열이기 때문에. 주어진 노드보다 큰 키가 가장 작은 노드는 그 후속 노드로 정의됩니다. BST에는 노드의 오른쪽 하위 트리 또는 조상에서 가장 적은 값을 갖는 노드 인 순차 후속 작업에 대한 두 가지 가능성이 있습니다. 그렇지 않으면 노드의 inorder 후속 작업이 존재하지 않습니다.
BST 알고리즘의 Inorder 후계자
root==NULL이면succ를NULL로 설정하고 반환합니다.root-> data<current-> data인 경우succ는current로current는current-> left로 표시됩니다.root-> data>current-> data인 경우current는current-> right로 표시됩니다.root-> data==current-> data및root-> right!=NULL,succ=minimum(current-> right)인 경우.succ를 반환합니다.
BST 일러스트레이션의 Inorder 후속 제품
3의 순차 후속자는4입니다.3에는 오른쪽 노드가 있고4는 오른쪽 하위 트리에서3보다 큰 가장 작은 노드이기 때문입니다.
4의 순차 후계자는5입니다.4에는 올바른 노드가 없기 때문에 해당 조상을 확인해야하며 그 중에서5는4보다 큰 가장 작은 노드입니다.
BST 구현의 Inorder 후계자
#include <iostream>
using namespace std;
class Node {
public:
int data;
Node *left, *right;
Node(int x) {
this->data = x;
this->left = this->right = NULL;
}
};
Node* insert(Node* root, int key) {
if (root == NULL) {
return new Node(key);
}
if (key < root->data) {
root->left = insert(root->left, key);
} else {
root->right = insert(root->right, key);
}
return root;
}
Node* getNextleft(Node* root) {
while (root->left) {
root = root->left;
}
return root;
}
void inorderSuccessor(Node* root, Node*& succ, int key) {
if (root == NULL) {
succ = NULL;
return;
}
if (root->data == key) {
if (root->right) {
succ = getNextleft(root->right);
}
}
else if (key < root->data) {
succ = root;
inorderSuccessor(root->left, succ, key);
} else {
inorderSuccessor(root->right, succ, key);
}
}
int main() {
int keys[] = {1, 5, 8, 2, 6, 3, 7, 4};
Node* root = NULL;
for (int key : keys) {
root = insert(root, key);
}
for (int key : keys) {
Node* prec = NULL;
inorderSuccessor(root, prec, key);
if (prec) {
cout << "Inorder successor of node " << key << " is " << prec->data;
} else {
cout << "No inorder Successor of node " << key;
}
cout << '\n';
}
return 0;
}
BST 알고리즘에서 Inorder Successor의 복잡성
시간 복잡성
- 평균 사례
평균적인 경우 BST에서 순서가 뒤 따르는 것을 찾는 시간 복잡도는 이진 검색 트리의 높이 순서입니다. 평균적으로 BST의 높이는O(logn)입니다. 형성된 BST가 균형 BST 일 때 발생합니다. 따라서 시간 복잡도는 [Big Theta] :O(logn)의 순서입니다.
- 베스트 케이스
최상의 경우는 트리가 균형 잡힌 BST 일 때 발생합니다. 삭제의 가장 좋은 경우 시간 복잡도는O(logn)순서입니다. 평균 케이스 시간 복잡성과 동일합니다.
- 최악의 경우
최악의 경우 루트에서 가장 깊은 리프 노드, 즉 트리의 전체 높이h로 이동해야 할 수 있습니다. 트리의 균형이 맞지 않는 경우 (예 : 치우친 경우) 트리의 높이가n이 될 수 있으므로 삽입 및 검색 작업의 최악의 경우 시간 복잡성은O(n)입니다.
공간 복잡성
알고리즘의 공간 복잡도는 재귀 호출에 필요한 추가 공간으로 인해O(h)입니다.
